

Welcome meeting Thursday September 7 2023 at 10am, room 4, Institut Fourier
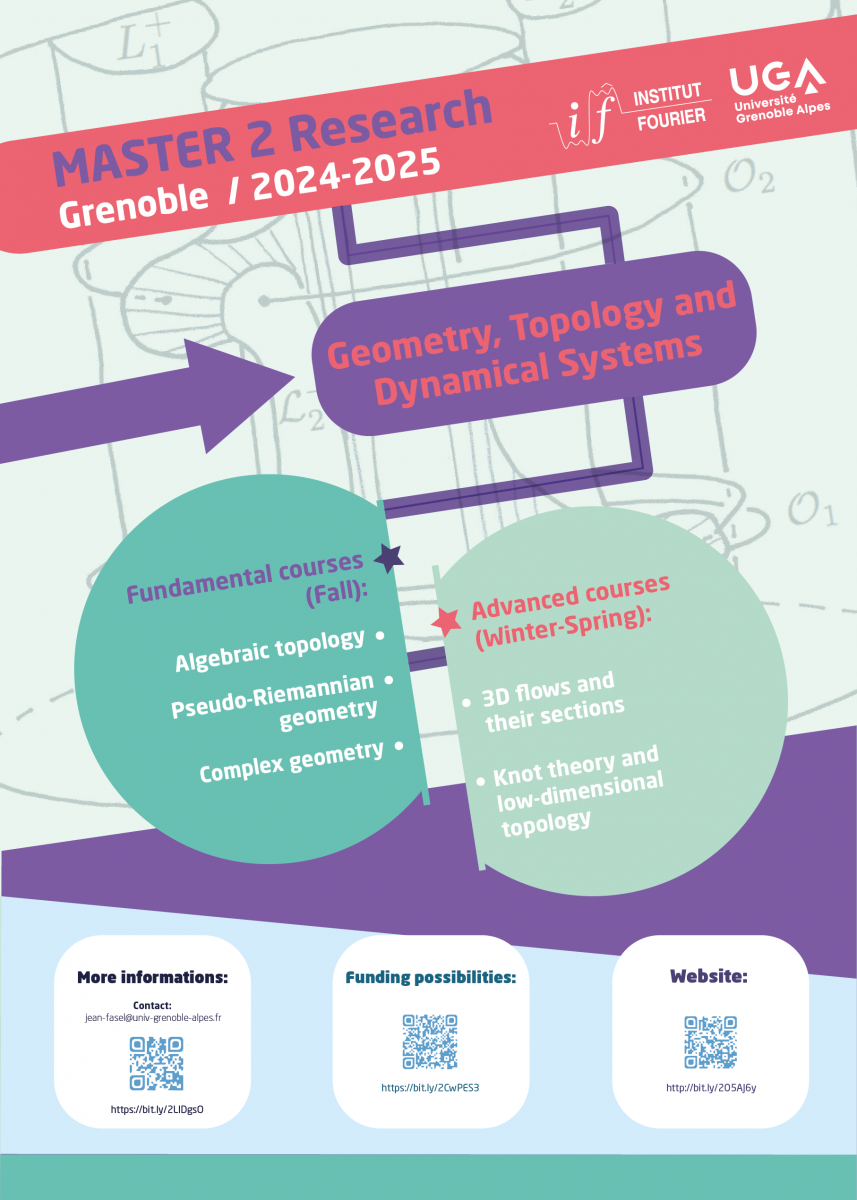 The Master 2 degree in fundamental mathematics (M2R) of the University of Grenoble Alpes offers each year a training in research in a selected topic, with possibilities to pursue in a PhD in the Institut Fourier (Grenoble), one of the French prominent institutes in pure mathematics. Courses are taught in English if non-french-speaking students are registered. The topic of the academic year 2023-24 is Geometrical aspects in probability. The topic of the academic year 2024-2025 will be Geometry, Topology and Dynamical Systems. Fundamental courses will give a comprehensive introduction to Algebraic Topolgy, (Pseudo-) Riemannian Geometry and Complex Differential Geometry. The more advanced courses will concern 3D flows and their sections as well as Knot theory and low-dimensional Topology.
The Master 2 degree in fundamental mathematics (M2R) of the University of Grenoble Alpes offers each year a training in research in a selected topic, with possibilities to pursue in a PhD in the Institut Fourier (Grenoble), one of the French prominent institutes in pure mathematics. Courses are taught in English if non-french-speaking students are registered. The topic of the academic year 2023-24 is Geometrical aspects in probability. The topic of the academic year 2024-2025 will be Geometry, Topology and Dynamical Systems. Fundamental courses will give a comprehensive introduction to Algebraic Topolgy, (Pseudo-) Riemannian Geometry and Complex Differential Geometry. The more advanced courses will concern 3D flows and their sections as well as Knot theory and low-dimensional Topology.
Applications to the Master 2 year will be open on this server (click) on February 21 2023.
All information about GRANTS and SCHOLARSHIPS are available in this page (click). CROUS Scholarships applications will also open soon on this page (click). We strongly recommend to apply quickly, because we experienced last year that CROUS seems to refuse the applications if they are made too late.
Contact : Jean.Fasel at univ-grenoble-alpes.fr (head of the program),
latifa.hamed-abdelouahab at univ-grenoble-alpes.fr (adminsitration)