



suivant: Power in /p and
monter: Computing in /p or
précédent: Euclidian quotient and euclidian
Table des matières
Index
Division in
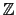 /p
/p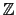 or in
or in
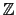 /p
/p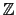 [x] : /
[x] : /
/ divides two integers in
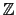 /p
/p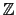 or
two polynomials A and B in
or
two polynomials A and B in
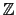 /p
/p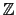 [x].
[x].
For polynomials, the result is the irreducible representant
of the fraction
 in
in
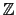 /p
/p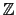 [x].
[x].
For integers in
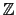 /p
/p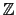 , input :
, input :
5%13/2% 13
Since 2 is invertible in
Z/13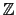 , we get the output :
, we get the output :
-4%13
For polynomials with coefficients in
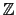 /p
/p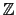 , input :
, input :
(2*x^2+5)%13/(5*x^2+2*x-3)%13
Output :
((6%13)*x+1%13)/((2%13)*x+2%13)
giac documentation written by Renée De Graeve and Bernard Parisse