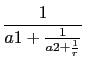



suivant: Transform a continued fraction
monter: Rationals
précédent: Simplification of a pair
Table des matières
Index
Continued fraction representation of a real : dfc
dfc takes as argument a real or a rational or a
floating point number a and an integer n
(or a real epsilon).
dfc returns the list of the continued fraction representation
of a of order n (or with precision epsilon i.e.
the continued fraction representation which
approachs a or evalf(a) with precision
epsilon, by default epsilon is the value of the epsilon
defined in the cas configuration with the menu
Cfg
 Cas Configuration).
Cas Configuration).
convert with the option confrac has a similar
functionnality: in that case
the value of epsilon is the value of the epsilon
defined in the cas configuration with the menu
Cfg
 Cas Configuration (see
1.21.23)
and the answer may be stored in an optionnal third argument.
Cas Configuration (see
1.21.23)
and the answer may be stored in an optionnal third argument.
Remarks
- If the last element of the result is a list, the representation is
ultimaltely periodic, and the last element is the period. It means
that the real is a root of an equation of order 2 with integer
coefficients.
- if the last element of the result is not an integer, it
represents a remainder r (
a = a0 + 1/.... + 1/an + 1/r). Be aware
that this remainder has lost most of it's accuracy.
If dfc(a)=[a0,a1,a2,[b0,b1] that means :
a =
a0 +

If dfc(a)=[a0,a1,a2,r] that means :
a =
a0 +
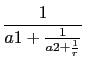
Input :
dfc(sqrt(2),5)
Output :
[1,2,[2]]
Input :
dfc(evalf(sqrt(2)),1e-9)
Or :
dfc(sqrt(2),1e-9)
Output :
[1,2,2,2,2,2,2,2,2,2,2,2,2]
Input :
convert(sqrt(2),confrac,'dev'
Output (if in the cas configuration epsilon=1e-9) :
[1,2,2,2,2,2,2,2,2,2,2,2,2]
and [1,2,2,2,2,2,2,2,2,2,2,2,2] is stored in dev.
Input :
dfc(9976/6961,5)
Output :
[1,2,3,4,5,43/7]
Input to verify:
1+1/(2+1/(3+1/(4+1/(5+7/43))))
Output :
9976/6961
Input :
convert(9976/6961,confrac,'l')
Output (if in the cas configuration epsilon=1e-9) :
[1,2,3,4,5,6,7]
and [1,2,3,4,5,6,7] is stored in l
Input :
dfc(pi,5)
Output :
[3,7,15,1,292,(-113*pi+355)/(33102*pi-103993)]
Input :
dfc(evalf(pi),5)
Output (if floats are hardware floats, e.g. for Digits=12) :
[3,7,15,1,292,1.57581843574]
Input :
dfc(evalf(pi),1e-9)
Or :
dfc(pi,1e-9)
Or (if in the cas configuration epsilon=1e-9) :
convert(pi,confrac,'ll')
Output :
[3,7,15,1,292]




suivant: Transform a continued fraction
monter: Rationals
précédent: Simplification of a pair
Table des matières
Index
giac documentation written by Renée De Graeve and Bernard Parisse